The Simplest Radical Form: Rules And Applications Explained
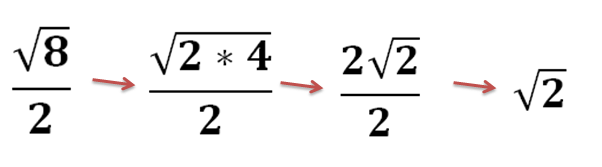
The Simplest Radical Form: Rules And Applications Explained. Discover more detailed and exciting information on our website. Click the link below to start your adventure: Visit Best Website. Don't miss out!
Table of Contents
Mastering the Simplest Radical Form: Rules and Applications Explained
Simplifying radical expressions can feel daunting, but understanding the simplest radical form is a crucial skill in algebra and beyond. This guide breaks down the rules and applications of simplifying radicals, empowering you to tackle even the most complex expressions with confidence. Whether you're a high school student tackling your math homework or a seasoned mathematician brushing up on fundamentals, this guide offers clear, concise explanations and practical examples.
What is the Simplest Radical Form?
The simplest radical form of a number is a way of expressing a radical (a square root, cube root, etc.) without any perfect square factors within the radicand (the number under the radical symbol). In essence, it's the most streamlined and efficient representation of the radical. For example, √12 is not in simplest radical form because 12 contains the perfect square factor 4 (12 = 4 x 3). The simplest radical form of √12 is 2√3.
Key characteristics of a radical in simplest form:
- No perfect square (or higher power) factors under the radical sign: This is the most fundamental rule.
- No fractions under the radical sign: Radicals should be simplified to eliminate fractions within the radicand.
- No radicals in the denominator: Rationalize the denominator to remove any radicals from the bottom of a fraction.
Rules for Finding the Simplest Radical Form
Simplifying radicals follows a set of straightforward rules. Let's explore them:
1. Prime Factorization: The Foundation
The first step in simplifying any radical is to find the prime factorization of the number under the radical sign. This breaks the number down into its prime components, allowing you to identify perfect squares (or cubes, etc.) easily.
Example: Let's simplify √72.
-
Find the prime factorization of 72: 2 x 2 x 2 x 3 x 3 = 2³ x 3²
-
Identify perfect squares: We see 2² and 3².
-
Simplify: √72 = √(2² x 3² x 2) = 2 x 3 √2 = 6√2
2. Simplifying nth Roots
The principle extends to roots beyond square roots. For cube roots (∛), you look for perfect cubes; for fourth roots (∜), you look for perfect fourths, and so on.
Example: Simplify ∛54
-
Prime factorization of 54: 2 x 3 x 3 x 3 = 2 x 3³
-
Identify perfect cube: 3³
-
Simplify: ∛54 = ∛(2 x 3³) = 3∛2
3. Rationalizing the Denominator
If you have a radical in the denominator of a fraction, you must rationalize it. This involves multiplying both the numerator and the denominator by a suitable expression to eliminate the radical from the denominator.
Example: Simplify 4/√2
-
Multiply the numerator and denominator by √2: (4 x √2) / (√2 x √2)
-
Simplify: (4√2) / 2 = 2√2
Applications of Simplest Radical Form
Simplifying radicals isn't just an abstract exercise; it has practical applications across various fields:
- Geometry: Calculating areas, volumes, and lengths often involves simplifying radicals.
- Physics: Many physical equations utilize radicals, requiring simplification for accurate calculations.
- Engineering: Structural design, circuit analysis, and other engineering disciplines rely on simplified radical expressions.
Mastering Radicals: Your Next Steps
Understanding and applying the simplest radical form is a cornerstone of mathematical proficiency. By mastering prime factorization and the rules of radical simplification, you'll significantly enhance your problem-solving skills across various mathematical domains. Start practicing with different examples, and soon, you’ll confidently navigate even the most challenging radical expressions. Need more help? Check out our [link to relevant resources/tutorials].
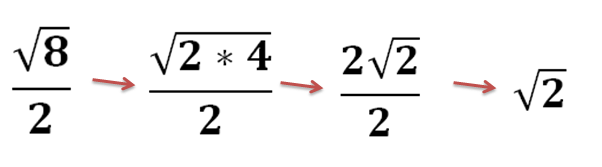
Thank you for visiting our website wich cover about The Simplest Radical Form: Rules And Applications Explained. We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and dont miss to bookmark.
Featured Posts
-
 Vivian Howards Diet And Fitness Plan How She Achieved Her Goals
Feb 05, 2025
Vivian Howards Diet And Fitness Plan How She Achieved Her Goals
Feb 05, 2025 -
 The Importance Of Sunlight As An Abiotic Factor In Ecology
Feb 05, 2025
The Importance Of Sunlight As An Abiotic Factor In Ecology
Feb 05, 2025 -
 Evolution Of The Human Forehead Size And Significance
Feb 05, 2025
Evolution Of The Human Forehead Size And Significance
Feb 05, 2025 -
 Do Trout Have Limbs Exploring Fish Anatomy
Feb 05, 2025
Do Trout Have Limbs Exploring Fish Anatomy
Feb 05, 2025 -
 Love Island Bombshell Samie Elishis Shocking Dm Reveal
Feb 05, 2025
Love Island Bombshell Samie Elishis Shocking Dm Reveal
Feb 05, 2025
Latest Posts
-
 Survival Evasion Planning Preparing For Unexpected Challenges
Feb 05, 2025
Survival Evasion Planning Preparing For Unexpected Challenges
Feb 05, 2025 -
 Is A Buffy The Vampire Slayer Reboot Even Needed
Feb 05, 2025
Is A Buffy The Vampire Slayer Reboot Even Needed
Feb 05, 2025 -
 Is Caillou Sick Understanding His Portrayal In The Show
Feb 05, 2025
Is Caillou Sick Understanding His Portrayal In The Show
Feb 05, 2025 -
 World Cancer Day 2025 The Latest On Urologic Cancers
Feb 05, 2025
World Cancer Day 2025 The Latest On Urologic Cancers
Feb 05, 2025 -
 Comparativa De Brocas Ncm Para Concreto Cual Elegir
Feb 05, 2025
Comparativa De Brocas Ncm Para Concreto Cual Elegir
Feb 05, 2025
